| Author: | Tsuyoshi Kato | ISBN: | 9789814635387 |
| Publisher: | World Scientific Publishing Company | Publication: | October 21, 2016 |
| Imprint: | WSPC | Language: | English |
| Author: | Tsuyoshi Kato |
| ISBN: | 9789814635387 |
| Publisher: | World Scientific Publishing Company |
| Publication: | October 21, 2016 |
| Imprint: | WSPC |
| Language: | English |
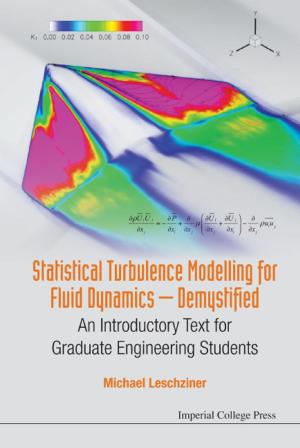
This book provides comprehensive analysis of dynamical systems in tropical geometry, which include the author's significant discoveries and pioneering contributions. Tropical geometry is a kind of dynamical scale transform which connects real rational dynamics with piecewise linear one presented by max and plus algebras. A comparison method is given which estimates orbits corresponding to different rational dynamics by reduction to the piecewise linear dynamics.
Both rational and piecewise linear dynamics appear in many important branches of mathematics. Tropical geometry can play a role or function to bridge between different subjects in mathematics. This book contains detailed accounts of basic strategy on how to apply tropical geometry to analysis in various mathematical subjects by presenting several applications which include: a rough classification of partial differential equations from the point of view of global behavior of solutions; construction of the infinite quasi-recursive rational dynamics, based on the automaton of the Burnside group by Aleshin-Grigorchuk; study on nearly periodicity of the pentagram map on the moduli space of the twisted polygons; spectral coincidence between lamplighter group in theory of automata groups and Box and ball systems corresponding to KdV equation in soliton theory.
This book is self-contained, and detailed accounts of theory of automata groups, BBS and the pentagram map are also included.
Contents:
-
Iterative Dynamics:
- Tropical Transform
- Dynamical Hierarchy
- Rational Perturbation of Dynamics in Two Variables
- Pentagram Map and Tropical Geometry
-
Iterative Dynamics:
- State Dynamics
- Automata Groups
- Stable State Dynamics
- Rational Burnside problem
- KdV Equation and Box-Ball Systems
- Spectral Similarity between BBS and Lamplighter Group
- Rough Comparison between Various Differential Equations
- Evolutional Dynamics
- Rough Analytic Relation on the Set of Partial Differential Equations
-
Mealy Type Dynamics:
- Hyperbolic System of Partial Differential Equations
- Analysis of Hyperbolic Mealy Systems
Readership: Graduate students and researchers in geometry.
Key Features:- This book is self-contained and many of the topics in this book are unique, which means they have never appeared elsewhere
This book provides comprehensive analysis of dynamical systems in tropical geometry, which include the author's significant discoveries and pioneering contributions. Tropical geometry is a kind of dynamical scale transform which connects real rational dynamics with piecewise linear one presented by max and plus algebras. A comparison method is given which estimates orbits corresponding to different rational dynamics by reduction to the piecewise linear dynamics.
Both rational and piecewise linear dynamics appear in many important branches of mathematics. Tropical geometry can play a role or function to bridge between different subjects in mathematics. This book contains detailed accounts of basic strategy on how to apply tropical geometry to analysis in various mathematical subjects by presenting several applications which include: a rough classification of partial differential equations from the point of view of global behavior of solutions; construction of the infinite quasi-recursive rational dynamics, based on the automaton of the Burnside group by Aleshin-Grigorchuk; study on nearly periodicity of the pentagram map on the moduli space of the twisted polygons; spectral coincidence between lamplighter group in theory of automata groups and Box and ball systems corresponding to KdV equation in soliton theory.
This book is self-contained, and detailed accounts of theory of automata groups, BBS and the pentagram map are also included.
Contents:
-
Iterative Dynamics:
- Tropical Transform
- Dynamical Hierarchy
- Rational Perturbation of Dynamics in Two Variables
- Pentagram Map and Tropical Geometry
-
Iterative Dynamics:
- State Dynamics
- Automata Groups
- Stable State Dynamics
- Rational Burnside problem
- KdV Equation and Box-Ball Systems
- Spectral Similarity between BBS and Lamplighter Group
- Rough Comparison between Various Differential Equations
- Evolutional Dynamics
- Rough Analytic Relation on the Set of Partial Differential Equations
-
Mealy Type Dynamics:
- Hyperbolic System of Partial Differential Equations
- Analysis of Hyperbolic Mealy Systems
Readership: Graduate students and researchers in geometry.
Key Features:- This book is self-contained and many of the topics in this book are unique, which means they have never appeared elsewhere